risevast.blogg.se - Algebra 1 geometric sequences

#Algebra 1 geometric sequences series#

The sequence below is an example of a geometric sequence because each term increases by a constant factor of 6. Each term of a geometric sequence increases or decreases by a constant factor called the common ratio. This would give us, which we could solve to get. The yearly salary values described form a geometric sequence because they change by a constant factor each year.

Also, many problems throughout the curriculum, including all multiple choice questions, are released items retrieved from ACT, New Visions, PARCC, PSAT, SAT, and TEA. Here is a Google Drive folder with every handout and another folder with an answer key for every handout.

represents the position of a term in the sequence.Įxample: To find the sum of we plug the following into the sum formula, : This curriculum is based on a schedule with 50 minute classes.

is the sum of the terms in the sequence.

The product contains a self-checking printable and digital activity for arithmetic and geometric sequences as a list of terms, as explicit formulas, as recursive formulas, in sequence notation and in function notation.

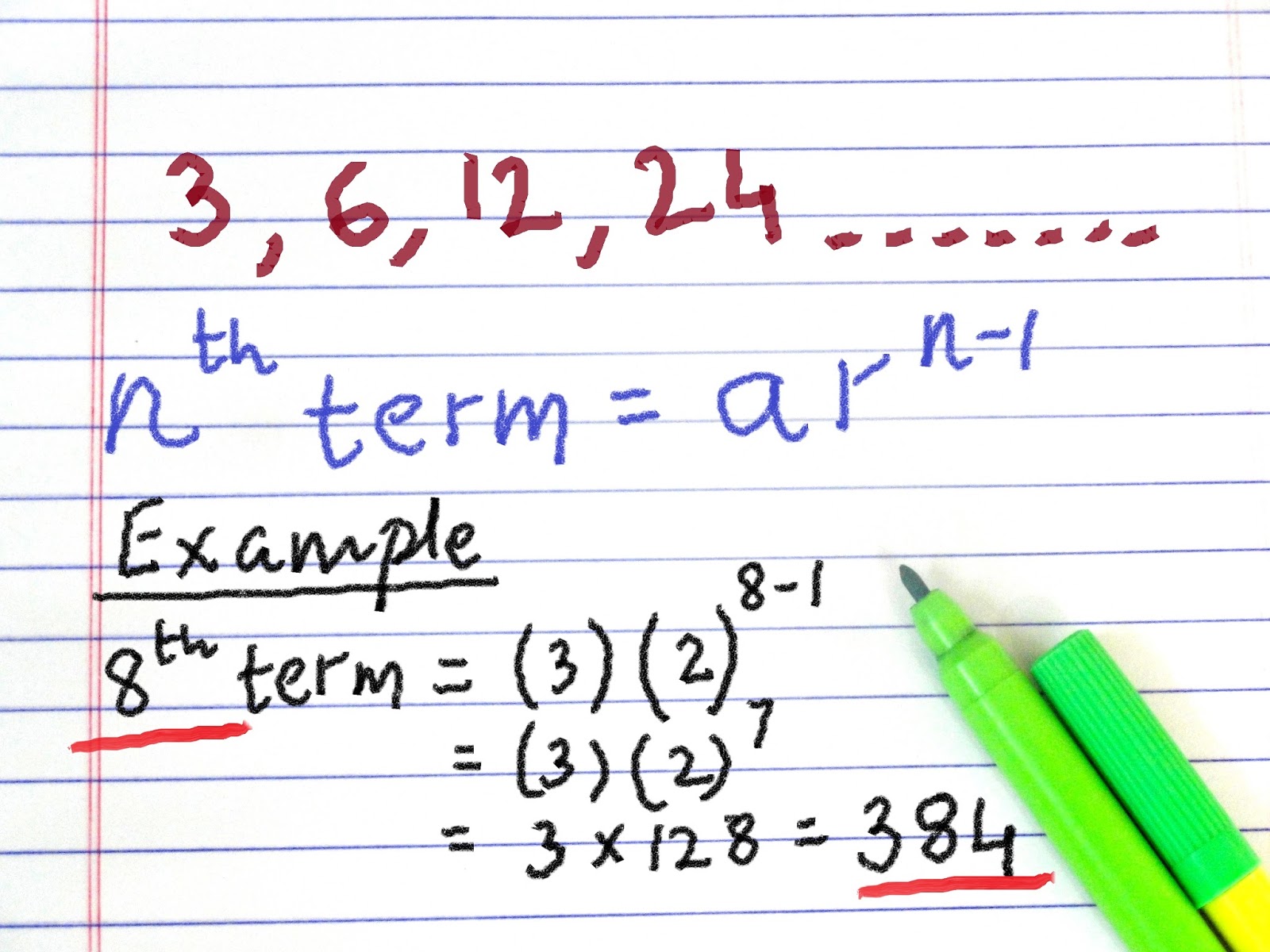
So, our sequence would be: Finding the sum of all the terms in a geometric sequence: Arithmetic and Geometric Sequences in Algebra 1 Self-Checking Activity. This would give us, which we could solve to get.

In which the last term is raised to the power of (because the first term is raised to the power of ).Įxample: To find the next term in which would be the 6th term, we would plug the following into the general term formula, : A sequence with number of terms, for example, would be written as:

represents the position of a term in the sequence. The fixed amount is called the common ratio, r, referring to the fact that the ratio (fraction) of second term to the.

Enter the keystrokes on a calculator and record the results in the table. Describing Calculator Patterns Work with a partner. Solve one-step and two-step linear equations: word problems. Įxample: if the first term of the sequence is and the common ratio is, then each successive term can be obtained by multiplying the previous term by 3, and the sequence will look like this:įinding any term ( ) in a geometric sequence: In a geometric sequence, the ratio between each pair of consecutive terms is the same. Model and solve linear equations using algebra tiles 1.1: Solving Equations 1.

represents the first term and is sometimes written as. If youre going on to Calculus, these are going to be important Remember that with arithmetic sequences we added something each time. For an arithmetic sequence with first term u1 and common difference d, the nth term is un u1 + (n 1) d.

The standard form of geometric sequences can be expressed as:

1, 2, 4, 8, 16, Algebra Name Arithmetic and Geometric Sequences. 2, 6, 18, 54, This is an increasing geometric sequence with a common ratio of 3. The factor by which each successive term is multiplied is called the common ratio because it is common to all of the terms in the set. Sometimes the terms of a geometric sequence get so large that you may need to express the terms in scientific notation rounded to the nearest tenth.

#Algebra 1 geometric sequences series#

In mathematics, a geometric series is the sum of an infinite number of terms that have a constant ratio between successive terms.A geometric sequence, also called a geometric series or geometric progression, is a set of numbers formed by multiplying each previous number in the set by a constant. The total purple area is S = a / (1 - r) = (4/9) / (1 - (1/9)) = 1/2, which can be confirmed by observing that the unit square is partitioned into an infinite number of L-shaped areas each with four purple squares and four yellow squares, which is half purple. Another geometric series (coefficient a = 4/9 and common ratio r = 1/9) shown as areas of purple squares.